PhD Thesis Defense | Jon Lasa Alonso

Space-time symmetries in classical and quantum electromagnetic scattering theory

December 1, 11:00

CFM Auditorium

Candidate: Jon Lasa Alonso

Supervisors:  Gabriel Molina Terriza and Aitzol García Etxarri

DOWNLOAD FULL THESIS

Summary

In this thesis, we propose a novel approach to study electromagnetic wave dynamics based on the systematic application of group theory. We show that this alternative approach leads to new insights on well-studied topics such as linear electromagnetic scattering theory or the emergence of the Kerker phenomena.

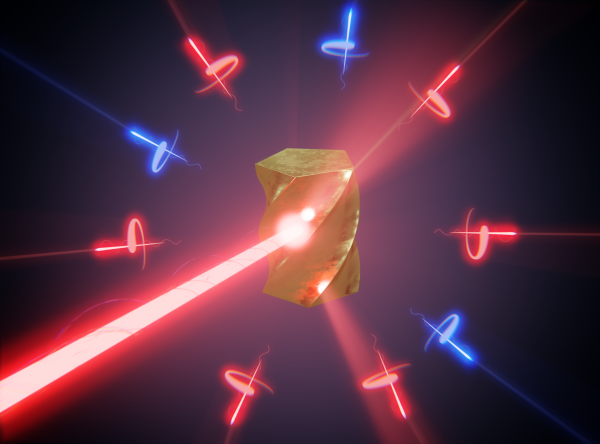
A laser beam impinges on a sample which, in turn, scatters waves of both helicities (blue and red arrows). Helicity components of the scattered field can be analyzed to obtain further information about the sample.

First of all, we show that the monochromatic version of the Riemann-Silberstein (RS) vector is associated with the unitary irreducible representations of the P(3,1) subgroup of the Poincaré group. Then, we show that its application to scattering problems elucidates many fundamental properties of linear electromagnetic samples. In this line, we show that losses preclude the existence of dielectric dual scatterers and that optical gain is a necessary condition to build antidual scatterers of any size and form. Also, we introduce the Single Characterization Angle (SCA) method that permits the optical characterization of cylindrical samples in favourable experimental conditions.

On the other hand, based on the monochromatic RS vector, we study the propagation of electromagnetic waves in inhomogeneous magnetic media. We show that systems made from different materials with the same refractive index are optimal for flipping the helicity of light. Moreover, we also identify a conserved quantity associated with this kind of environments, i.e. the square of linear momentum, whose emergence can be well-understood in terms of the P(3,1) subgroup of the Poincaré group. Finally, we provide an alternative interpretation of the Kerker phenomena based on group theoretical arguments.

Our last contribution focuses on the quantum scattering of multiphoton states of light with cylindrical samples. We show that there are multiphoton states of light, i.e. the so-called symmetry-protected states, which are left invariant in the interaction with this kind of scatterers. We propose their use in the construction of decoherence-free subspaces.