Validity of effective potentials in crowded solutions of reversible network-forming polymers

By combining large-scale simulations and liquid state theory, we¡¡the authors find that the effective fluid approach provides an excellent description of the structural correlations in reversible polymer networks even in highly crowded conditions. This is a very unusual result in macromolecular solutions, and it is a consequence of the molecular size being unperturbed through reversible intermolecular bonding. Moreover, this results suggests that experimental interpenetrated networks are out-of-equilibrium states where demixing is prevented through dynamic arrest.

The effective potential between two macromolecules separated by a given distance is the free energy needed to bring them from infinity to that distance. Unlike in hard-core colloids, the free energy cost for full interpenetration is finite because their centers-of-mass can coincide in space. This cost strongly depends on their topology and internal deformability, typically varying between a few and tens of times the thermal energy. Averaging out the molecular degrees of freedom and keeping a few relevant coordinates (usually the centers-of-mass) reduces the system to an effective fluid of ultrasoft particles interacting through the effective potential. This methodology allows not only for simulating much larger scales than in the all-monomers system but also for the treatment of the system by methods from liquid state theory, producing a powerful tool to predict large-scale organization and phase behavior. This approximation works below and even slightly above the overlap concentration, (the concentration at which the mean intermolecular distance is of the order of the unperturbed molecular size). However, it fails dramatically far above the overlap concentration when many-body effects become a dominant contribution (shrinkage of the molecular size under crowding being a well-known manifestation of them).

By means of large-scale simulations, Paciolla et al. have systematically derived effective potentials for polymers with reversible bonds, which in crowded conditions can form dynamic networks with viscous flow and self-healing properties. The authors have compared the static correlations in the effective fluid and in the all-monomers system. Rather surprisingly, an excellent agreement is found even far above the overlap concentration. This is consistent with the fact that shrinking is highly prevented by forming intermolecular bonds with neighboring chains, which unlike in crowded polymers without bonds, makes the conformations weakly sensitive to crowding and many-body effects basically contribute as a flat energy landscape.

Finally, this work explored the possibility of forming two interpenetrated networks in a polymer mixture where the reactive sites of the two components are orthogonal; i.e., intermolecular bonds only occur between chains of the same component. In agreement with the energetic penalty found for the effective cross-interaction potential and the phase diagram predicted for the effective fluid, no interpenetrated networks are found in the all-monomers solutions. Instead, the two components phase separate (demixing), as can be seen in the figure. This result suggests that experimental interpenetrated networks, where both the bond lifetimes and the intrinsic diffusion times of the polymers are much longer than in the simulations, are actually kinetically trapped states with large entropic barriers impeding the relaxation to the equilibrium demixed state.

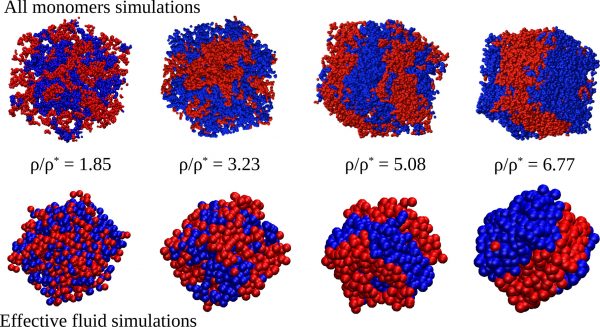
Figure: Snapshots of the all-monomers system (AM, upper row) and the effective fluid (EF, bottom row) of a binary mixture of polymer chains with orthogonal reversible bonding. The beads represent the actual monomers in the AM case and the effective ultrasoft particles in the EF. Molecules belonging to different components of the mixture are represented by different colors. Demixing is evident in both the AM and EF simulation. Concentrations (ρ) are given in terms of the overlap concentration (ρ*) and correspond to highly crowded conditions.