Theory of the Supercurrent Diode Effect in Rashba Superconductors with Arbitrary Disorder

The diode effect in superconductors attracts great interest for its promising applications in superconducting electronics. Furthermore, this effect is predicted to be observed in helical superconductors, i.e., superconductors with strong spin-orbit coupling and an exchange field.

Almost all previous theoretical works only considered ballistic transport in ideally clean superconductors. In this work, the authors demonstrate how the diode effect survives disorder and can be expected to be observed in real materials.

The interplay between superconductivity, spin-orbit coupling (SOC), and a Zeeman field leads to a variety of magnetoelectric effects widely studied in the past years. One of these effects is a nonreciprocal charge transport due to the breaking of time-reversal and inversion symmetries. Nonreciprocity manifests, for example, in the supercurrent in noncentrosymmetric superconducting structures and in Josephson junctions. The critical current depends on the direction of the current flow: by tuning the amplitude of the current between the two critical values the system will behave as a normal conductor in one direction and as a superconductor in the other. Such systems are being suggested as superconducting diodes with potential applications in low-power logic circuits.

All previous theoretical works assume ideally pure superconducting structures and disregard the effect of disorder. However, disorder is unavoidable in realistic structures, and therefore it is important to understand how it affects the supercurrent diode effect. In this work, the team establishes the microscopic theory of the supercurrent diode effect in disordered two-dimensional Rashba superconductors.

These results elucidate the mechanisms leading to the diode effect, and show how it evolves in the full range of all relevant system parameters: SOC, magnetic field, temperature, and disorder. Namely, the effect stems from the comp

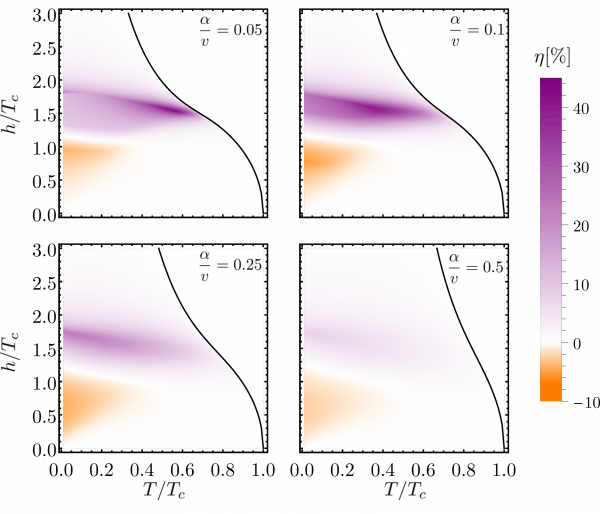
Figure: The diode quality factor calculated for every point in the Zeeman field (h)− Temperature (T) phase diagram at different strengths of spin-orbit coupling.

etition between two helical bands in a Rashba super- conductor, which prefers opposite modulation vectors of the superconducting order parameter when magnetic field is applied. Both magnetic field and SOC are required for the diode effect; however, if either is too strong, the band competition ceases as one helical band begins to dominate, leading to the suppression of the effect. This means that a substantial diode effect exists only for some optimal magnetic field and SOC. Disorder further complicates this picture, as it introduces mixing of the two helical bands. illic et al. discuss optimal parameter regimes where the effect is strongest and establish that the effect persists even at strong disorder. Moreover, this work shows that the sign of the rectification changes by increasing the disorder. The change of sign can be related to the change of nature of the helical phase.